initialplot
Plot initial condition response of dynamic system
Description
The initialplot function plots the initial condition response
of a dynamic system
model and returns an
InitialPlot chart object. To customize the plot, modify the properties of
the chart object using dot notation. For more information, see Customize Linear Analysis Plots at Command Line.
To obtain initial condition response data, use the initial function.
Creation
Syntax
Description
ip = initialplot(sys,IC)sys and
returns the corresponding chart object.
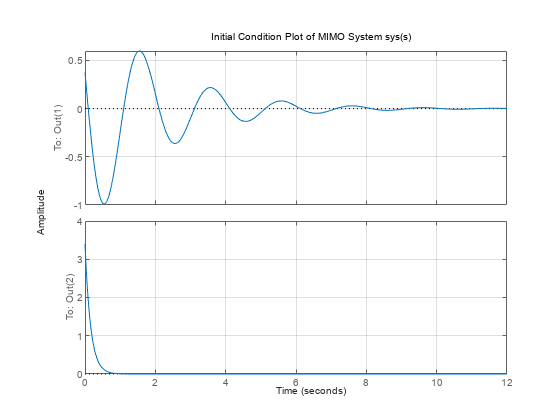
If sys is a multi-input, multi-output (MIMO) model, then the
initialplot function creates a grid of plots with each plot
displaying the initial condition response of one input-output pair.
If sys is a model with
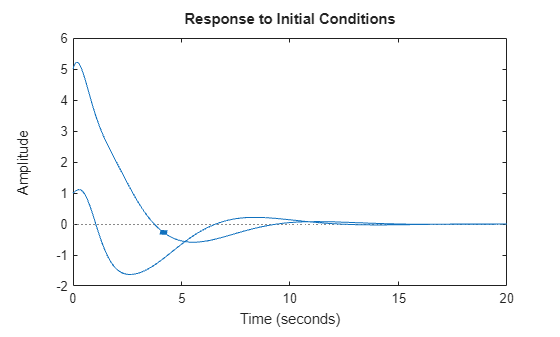
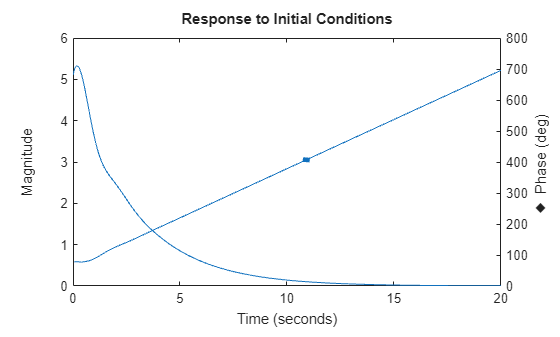
complex coefficients, then the plot shows both the real and imaginary components of the
response on a single axes and indicates the imaginary component with a diamond marker.
You can also view the response using magnitude-phase and complex-plane plots. (since R2025a)
ip = initialplot(___,t)t. You can
use t with any of the input argument combinations in previous
syntaxes. To define the time steps, you can specify:
The final simulation time using a scalar value.
The initial and final simulation times using a two-element vector. (since R2023b)
All the time steps using a vector.
ip = initialplot(___,plotoptions)plotoptions. Settings you specify in
plotoptions override the plotting preferences for the current
MATLAB® session. This syntax is useful when you want to write a script to generate
multiple plots that look the same regardless of the local preferences.
ip = initialplot(parent,___)Figure or TiledChartLayout, and sets the
Parent property. Use this syntax when you want to create a plot
in a specified open figure or when creating apps in App Designer.
Input Arguments
Properties
Object Functions
addResponse | Add dynamic system response to existing response plot |
Examples
Tips
Plots created using
initialplotdo not support multiline titles or labels specified as string arrays or cell arrays of character vectors. To specify multiline titles and labels, use a single string with anewlinecharacter.initialplot(sys) title("first line" + newline + "second line");